Принципы контроля наноматериалов для разработки стандартов безопасности на примере выявления закономерностей наноструктурирования в системах CayLa1-yF3-y и LaxCa1-xF2+x
© С.К. Максимов, К.С. Максимов
Московский государственный инсти тут электронной техники (Технический университет), Москва, Зеленоград
E-mail: email hidden; JavaScript is required
Поступило в Редакцию 21 августа 2008 г.
Сформулированы общие принципы экологического контроля наноматериалов и на основе закономерностей наноструктурирования в CayLa1-yF3-y и LaxCa1-xF2+x указана специфика этого контроля для материалов, являющихся растворами.
PACS: 61.46.Df
Производственный контроль должен, во-первых, обеспечить качество продукции, во-вторых, ограничить риски, связанные с технологиями, для окружающей среды, в первую очередь, для живой природы (экологический контоль). Однако в публикациях, посвященных технологиям наноматериалов, и даже в патентах, устанавливающих закономерности их производственного контроля, рассматривается только сторона, связанная с контролем качества (например, [1]).Экологический контроль основывается на стандартах безопасности (например, в химических производствах на предельно допустимых концентрациях веществ), однако для нанотехнологий принципы стандартов безопасности только дискутируются [2]. Поэтому мы делаем упреждающие попытки сформулировать принципы экологического контроля, исходя из трех факторов: а) механизмов, определяющих реакции на контакты между веществами, на молекулярном уровне, б) специфической термодинамики нанокристаллов, в) особенностей технологий, всегда предусматривающих допуски на параметры продуктов.
Реакции веществ на контакты друг с другом контролируются поверхностными функциональностями, т.е., способностями к адсорбции и управлению реакциями в адсорбированных слоях [3]. Поверхностная функциональность определяется электростатическим потенциалом поверхности, зависящим от структурно-морфологических характеристик: атомной и дефектной структур и индексов плоскостей контакта; у кристаллов алмаза этот потенциал положителен для {001} и отрицателен для {111} [4]. Одна и та же молекула по-разному реагирует на контакты с одним и тем же веществом в зависимости от структуры и морфологии контактной поверхности. Вклад поверхностной энергии в химпотенциал возрастает при уменьшении размеров кристаллов, и для наноразмерных частиц (с размером d < 100 nm) роль поверхностной энергии может быть соизмеримой с ролью энергии объемной, поэтому равновесные структура и морфология наночастиц могут отличаться от таковых для массивных форм. Например, при уменьшении размеров фазовое равновесие рутил/анатаз для кристаллов TiO2 сдвигается в сторону анатаза [3], а для кристаллов TiN их равновесный габитус трансформируется через ряд промежуточных форм от октаэдрического, ограненного по {111}, к гексаэдрическому, ограненному по {001} [5]. Переход к наноразмерам меняет поверхностную функциональность не только количественно, но и качественно. Вариации размеров в нанообласти — способ управления поверхностной функциональностью материалов.
Экологическая безопасность не может основываться на контроле только одного параметра, например суммарной площади поверхностей [2], по трем причинам. Во-первых, в нанообласти структурно-морфологические характеристики связаны термодинамически и при изменении каждой из них соответственно изменяются остальные. Причем габитус не может характеризоваться внешней формой, при его определении должна учитываться кристаллография огранки. Во-вторых, эти характеристики связаны функционально: при одних и тех же размерах и фазе кристаллы с разными габитусами имеют разные функциональности, и наоборот. В-третьих, они связаны методически: например, невозможно проиндицировать грани, если не определить их ориентации относительно кристаллографических осей. Экологический контроль должен основываться на триединой, неразрывной комбинации трех характеристик: размера, фазы, габитуса. Обязательность триединого контроля связана также с тем, что связи между структурно-морфологическими характеристиками имеют сложный характер и зависят также от других факторов, определяющих энергетику поверхностей, например, от поверхностно-активных веществ и примесного состава кристаллов.
В производстве невозможно получить частицы одного размера. Массивы, в которых 90–95% частиц имеют размеры, отличающиеся на 10%, рассматриваются как монодисперсные, хотя у остальных размеры могут варьироваться от 1 до 100 nm [1]. Однако в минорных фракциях могут иметься частицы с разными структурно-морфологическими формами. Материал в целом может не быть токсичным, но его минорные фракции могут влиять на метаболизм, что может сказаться через десятки лет. Особенно опасны массивы с характерными размерами <50 nm, для которых в диапазоне 1–50 nm может возникать несколько структурно-морфологических форм. Например, ZrO2 имеет модификации с моноклинной (m-ZrO2) и тетрагональной (t-ZrO2) структурами, и у каждой модификации существуют свои гексаэдрические, октаэдрические, дипирамидальные, а также переходные формы. Поэтому для ZrO2 даже вариации размеров в 10% в интервале 3–30nm могут вызывать принципиальные изменения поверхностных функциональностей [6]. Экологический контроль должен быть основан на выделении фракций с идентичными и/или близкими структурно-морфологическими характеристиками и проведении дальнейших контрольных операций, в том числе in vitro и in vivo, отдельно для каждой фракции.
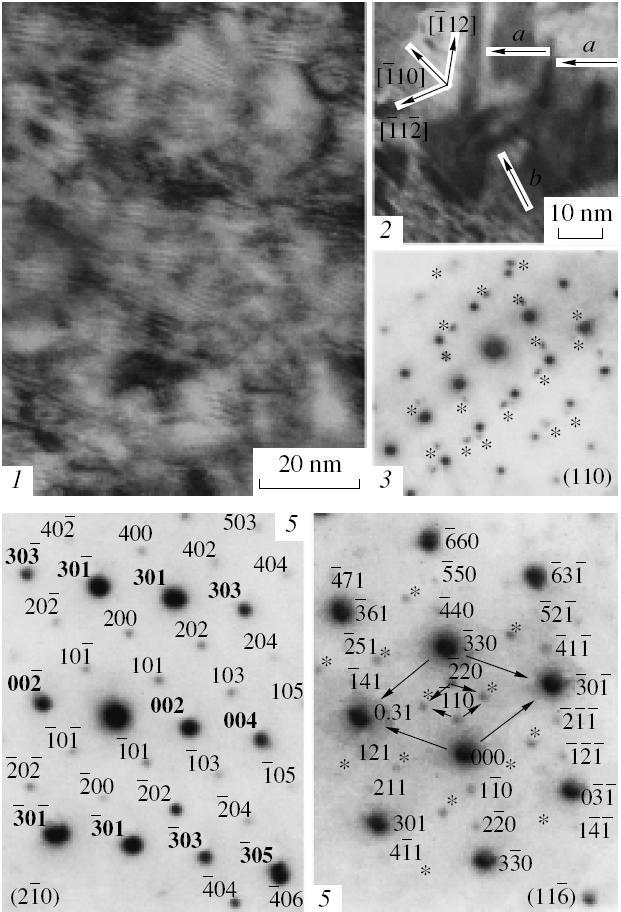
Структурно-морфологические характеристики наночастиц на основе растворов могут иметь специфические особенности, что следует из закономерностей преципитации в CaF2–LaF3. Преципитаты возникают в CayLa1-yF3-y при синтезе [1] и в LaxCa1-xF2+x при отжиге (рисунок, врезки 1 – 5).
Врезки 1, 2 и 3 отвечают проекции [110] для кубического LaxCa1-xF2+x. На врезке 1 объекты с осциллирующим контрастом — изображения двойников. На врезке 2 преципитат и порождаемые им двойники: a — двойники с плоскостью залегания ![]() , b — с плоскостью
, b — с плоскостью ![]() у преципитата развиты грани {111} и в меньшей степени — {001}. На врезке 3 наблюдаются матричные и двойниковые отражения (двойниковые отражения помечены звездочками),но отражения преципитатов отсутствуют. Электронограммы CayLa1-yF3-y на врезках 4, 5.
у преципитата развиты грани {111} и в меньшей степени — {001}. На врезке 3 наблюдаются матричные и двойниковые отражения (двойниковые отражения помечены звездочками),но отражения преципитатов отсутствуют. Электронограммы CayLa1-yF3-y на врезках 4, 5.
На врезке 4 индексы сверхструктурных рефлексов указаны петитом. Врезка 4 отвечает проекции ![]() ; сверхструктурные рефлексы 101, 202, 404,
; сверхструктурные рефлексы 101, 202, 404, ![]() ,
, ![]() и пр. лежат на правлениях <101>, а сверхструктурные — 200, 400 и т. д., тождественные в тригональной решетке отражениям
и пр. лежат на правлениях <101>, а сверхструктурные — 200, 400 и т. д., тождественные в тригональной решетке отражениям ![]() ,
, ![]() , …, — на направлении [100]. Направление порядка <101> требует границ со смещениями <101>. Врезка 5 — проекция
, …, — на направлении [100]. Направление порядка <101> требует границ со смещениями <101>. Врезка 5 — проекция ![]() ; отражения, помеченные звездочками и связанные только со сверхструктурными рефлексами, не индицируются в решетке CayLa1-yF3-y, стрелками указаны направления смещений <301>. Расстояния между сверхструктурными и несовместными рефлексами отвечают = 1.9 Å-1
; отражения, помеченные звездочками и связанные только со сверхструктурными рефлексами, не индицируются в решетке CayLa1-yF3-y, стрелками указаны направления смещений <301>. Расстояния между сверхструктурными и несовместными рефлексами отвечают = 1.9 Å-1
В LaxCa1-xF2+x они имеют тетрагонально искаженную решетку CaF2 с упорядоченным распределением ионов Ca+2 и La+3 по позициям катионной подрешетки [7], а в CayLa1-yF3-y – искаженную тригональную решетку LaF3, в которой вдоль направлений [001], [101] и [102] закономерно чередуются ион Ca+2 и 2 иона La+3 (врезка 2), что подразумевает состав La2CaF8 [8]. Упорядоченные фазы в системе CaF2 – LaF3 не известны, и согласно диаграмме состояния CaF2 – LaF3 при распаде пересыщенного раствора на основе CaF2 должны возникать преципитаты со структурой LaF3, а в растворенаоснове LaF3 – преципитаты со структурой CaF2 (ктому же содержание CaF2 в растворе на основе LaF3 не превышает 3 mol.%, чему противоречит состав La2CaF8) [9]. Преципитаты в LaxCa1-xF2+x имеют кубооктаэдрический габитус с преимущественным развитием граней {111} (врезка 2), что не соответствует закономерностям огранки преципитатов в кубической матрице [10]. Их габитус отражает энергетику границы раздела преципитат/матрица, обусловленную характерными искажениями кубической решетки при упорядочении по типу CuAu. Размеры преципитатов также определяются их структурой [7], т. е., структура и морфология нанопреципитатов подчиняется закономерностям, выявленным для изолированных нанокристаллов. Структурирование отражает минимизацию поверхностной энергии, и это правило не зависит от того, является ли поверхность свободной границей кристалла или границей между двумя твердыми фазами.
Релаксация напряжений вокруг преципитатов в LaxCa1-xF2+x вызывает двойникование. Двойники покрывают все поле зрения (врезка 1), что отражает высочайшую плотность преципитатов. Но на электронограммах от объемов ≈ 0.02 µm3 сверхструктурные рефлексы отсутствуют (врезка 3), и этому соответствует почти полное отсутствие изображений преципитатов на снимках, которые в лучшем случае лишь угадываются (врезка 1). Дифракционные картины отражают порядок, осредненный по рассеивающему объему, и отсутствие проявлений преципитации может быть связано с антифазными доменами, возникновение которых обусловлено стохастическим характером процесса упорядочения. Однако доменная структура частиц с размерами <20 nm означает рост поверхностной энергии и, на первый взгляд, противоречит самому принципу наноструктурирования, а следовательно, требует доказательств.
При отклонениях от точных проекций доменная структура ведет к возникновению сателлитов вблизи матричных рефлексов [8], что выявлено также для кубического LaxCa1-xF2+x [11]. Однако сателлиты могут также отражать двойникование, и для однозначного доказательства доменного строения были изучены закономерности электронограммы преципитатов LaxCa1-xF2+x с тригональной решеткой. Переход из домена в домен (при последовательном рассеянии на доменах) связан с фазовым сдвигом, зависящим от вектора смещения на междоменных границах. В упорядоченной фазе CayLa1-yF3-y существуют границы со смещениями <101>, и последовательная дифракция на разных доменах должна приводить к появлению рефлексов с нецелочисленными индексами (несовместных отражений) [8]. Для выявления доменов в преципитатах удобна проекция ![]() , для которой
, для которой ![]() является направлением с рядами сверхструктурных отражений (врезка 5). Направления <101> проецируются на плоскости
является направлением с рядами сверхструктурных отражений (врезка 5). Направления <101> проецируются на плоскости ![]() на направления <301>. Поэтому несовместные рефлексы, обусловленные сдвигами вдоль <101>, должны быть смещены относительно сверхструктурных рефлексов вдоль направлений <301> на расстояния, равные |g101| x cos σ ,где σ —уголмежду направлениями [301] и [101], равный 24.8º. В CayLa1-yF3-y возможны домены 3 типов, в которых Ca+2 и La+3 в катионной подрешетке чередуются как LaLaCa, LaCaLa и CaLaLa и вектора смещения на антифазных границах имеют два значения <101> и
на направления <301>. Поэтому несовместные рефлексы, обусловленные сдвигами вдоль <101>, должны быть смещены относительно сверхструктурных рефлексов вдоль направлений <301> на расстояния, равные |g101| x cos σ ,где σ —уголмежду направлениями [301] и [101], равный 24.8º. В CayLa1-yF3-y возможны домены 3 типов, в которых Ca+2 и La+3 в катионной подрешетке чередуются как LaLaCa, LaCaLa и CaLaLa и вектора смещения на антифазных границах имеют два значения <101> и ![]() , и возникают два ряда несовместных отражений, расположенных параллельно и симметрично относительно
, и возникают два ряда несовместных отражений, расположенных параллельно и симметрично относительно ![]() [8,12].
[8,12].
Степень порядка в LaxCa1-xF2+x изменяется от практически полного беспорядка до почти идеального La2CaF8, т.е. La2CaF8 — не соединение, а упорядоченное состояние в растворе CaF2 – LaF3 (как и упорядоченная фаза с кубической решеткой) [8,12]. Электронограмма 2,b содержит ряды несовместных отражений, расположенных по обе стороны и симметрично относительно ![]() . Несовместные отражения удалены от сверхструктурных вдоль <301> на 2.1Å-1 x cos 24.8º = 1.9 Å-1 (где 2.1 Å-1
. Несовместные отражения удалены от сверхструктурных вдоль <301> на 2.1Å-1 x cos 24.8º = 1.9 Å-1 (где 2.1 Å-1 ![]() |g101|). Электронограмма отвечает закономерностям предыдущего абзаца. La2CaF8 является не только упорядоченным, но также имеет антифазные домены. И упорядочение, и формирование антифазных доменов вписываются в представления о термодинамике нанокристаллитов. Упорядочение обеспечивает регулярность строения поверхности и соответственно минимизацию поверхностной энергии. Доменная структура обусловлена спонтанностью возникновения центров порядка. Энергия антифазных границ не велика и практически не влияет на диффузионный перенос из домена в домен. Поэтому в „обычных условиях“ этот перенос вызывает движение доменных границ, и „поедание“ одних доменов другими. Однако в нанокристаллах упругое взаимодействие антифазных границ с поверхностью может стабилизировать ранние стадии доменной структуры. Упорядочение, наличие антифазных доменов и степень порядка должны существенно влиять на функциональность наночастиц на основе растворов. Их необходимо включить в обязательный круг структурно-морфологических характеристик, контролируемых для этих наночастиц, особенно в связи с латентностью дифракционных проявлений упорядочения, связанной с наличием антифазных доменов.CayLa1-yF3-y and LaxCa1-xF2+x
|g101|). Электронограмма отвечает закономерностям предыдущего абзаца. La2CaF8 является не только упорядоченным, но также имеет антифазные домены. И упорядочение, и формирование антифазных доменов вписываются в представления о термодинамике нанокристаллитов. Упорядочение обеспечивает регулярность строения поверхности и соответственно минимизацию поверхностной энергии. Доменная структура обусловлена спонтанностью возникновения центров порядка. Энергия антифазных границ не велика и практически не влияет на диффузионный перенос из домена в домен. Поэтому в „обычных условиях“ этот перенос вызывает движение доменных границ, и „поедание“ одних доменов другими. Однако в нанокристаллах упругое взаимодействие антифазных границ с поверхностью может стабилизировать ранние стадии доменной структуры. Упорядочение, наличие антифазных доменов и степень порядка должны существенно влиять на функциональность наночастиц на основе растворов. Их необходимо включить в обязательный круг структурно-морфологических характеристик, контролируемых для этих наночастиц, особенно в связи с латентностью дифракционных проявлений упорядочения, связанной с наличием антифазных доменов.CayLa1-yF3-y and LaxCa1-xF2+x
Список литературы
[1] Bawendi M.G., Jensen K.F. Inventory control. USA Patent N 6, 774, 361, 10.08.2004.
[2] Katao Kazuo. // Clean Technologies and Environmental Policy. 2006. V. 8. N 4. P. 251–259.
[3] Zapol P., Curtiss L.A. // J. of Computational and Theoretical Nanoscience. 2007. V. 4. N 2. P. 222–230.
[4] Barnard A.S., Russo S.P., Snook I.K. // Diamond and Related Materials. 2003. V. 12. N 10. P. 1867–1872.
[5] Barnard A.S. // J. of Computational and Theoretical Nanoscience. 2004. V. 1. N 3. P. 334–339.
[6] Barnard A.S., Yered la R.R., Xu H. // Nanotechnology. 2006. V. 17. N 6. P. 3039–3047.
[7] Максимов С.К., Максимов К.С. // Неорганические материалы. 2007. Т. 43. №5.С.626–631.
[8] Максимов С.К. // Докл. РАН. 2007. Т. 416. № 1. С. 43–46.
[9] Sobolev B.P. The Rare Earth Trifluorides. Part 1. The High Temperature Chemistry of the Rare Earth Trifluorides. Institut d’Estudis Catalans. Barcelona, 2000. 520 p.
[10] Onaka S. // Philos. Mag. Lett. 2006. V. 86. P. 175–183.
[11] Максимов С.К., Эрреро П., Соболев Б.П. // Письма в ЖТФ. 2004. Т. 30. В. 23. С. 15–23.
[12] Максимов С.К., Максимов К.С. // Неорганические материалы. 2008. Т. 44. №8.
(1) Образцы для исследований были предоставлены Соболевым Б.П., ИК РАН.

 English
English